Power set
Consider a bag with three types of balls: red, blue and green. The power set is the set of all balls, the empty set, every ball individually, and combinations of the balls. That is, the set of all subsets of a set is called the power set. The power set is a fundamental concept in set theory that refers to the set of all possible subsets of a given set, including the empty set and the set itself. Understanding power sets is essential for building a strong foundation in topics like functions, relations, logic, and combinatorics. Whether you're studying discrete mathematics, preparing for competitive exams, or exploring basic set operations, mastering the concept of a power set is crucial. In this article, we will explain the definition, notation, properties, and examples of Power Sets in a clear and comprehensive manner.
This Story also Contains
- What is a Power Set?
- Power Set Examples with Solutions
- Power Set in Mathematics and Real-Life Applications
- Difference Between Set and Power Set
- Solved Examples Based on Power Set
- List of Topics Related to Power Set
- NCERT Resources
- Practice Questions on Power Sets
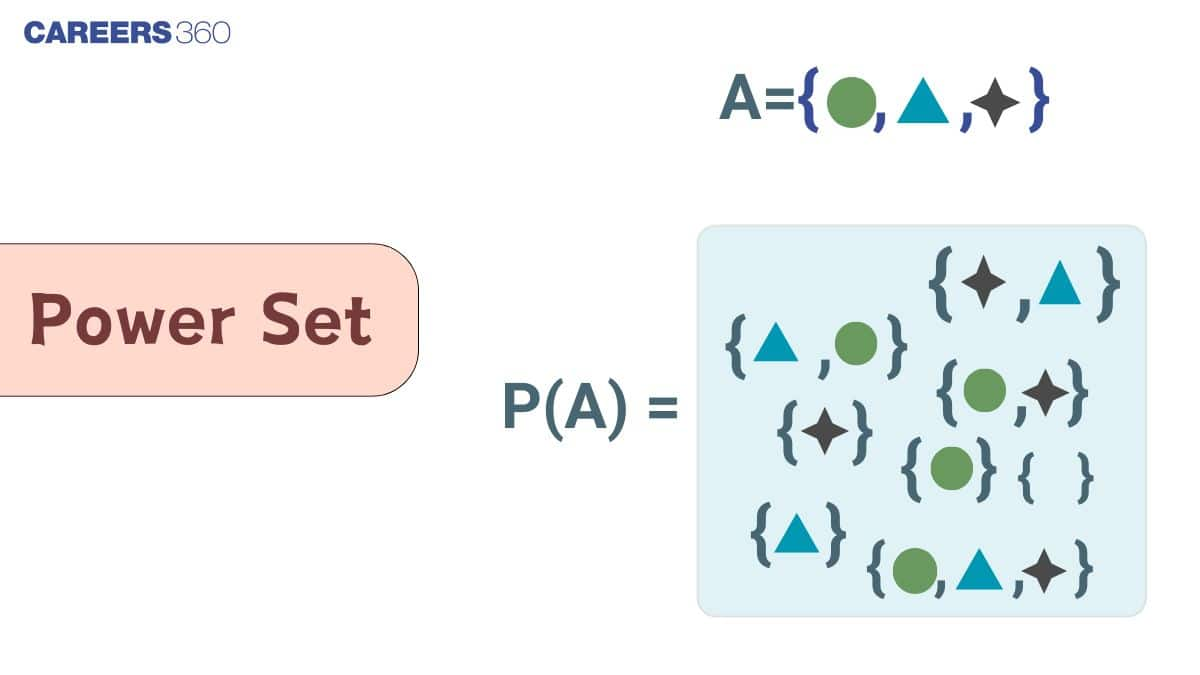
What is a Power Set?
The set of all subsets of a set $S$ is called the power set of$S$. It is denoted by $P(S)$. It also includes the empty set and the set itself. The power set always contains $2^n$ elements, where $n$ is the number of elements in the original set.
Power Set Definition: The collection of all subsets of a set $A$ is called the power set of $A$. It is denoted by $P(A)$.
Power Set Example: Let $\mathrm{A}=\{\mathrm{a}, \mathrm{b}, \mathrm{c}\}$, then $P(A)=\{\phi,\{a\},\{b\},\{c\},\{a, b\},\{b, c\},\{c, a\},\{a, b, c\}\}$
Cardinality of Power Set
The number of distinct elements in a finite set $A$ is called the Cardinal number or cardinality of set and it is denoted by $A$ and it is denoted by $n(A)$.
The power set formula to find the cardinality(number of elements) of the power set of any set $A$ is $2^n$ where $n$ is the number of elements in $A$.
Eg. Let $\mathrm{A}=\{\mathrm{a}, \mathrm{b}, \mathrm{c}\}$. The cardinality of the set $A$ is $3$. The cardinality of the power set of $A$, $P(A)$ is $2^n = 2^3 = 8$.
Properties of Power Set
The properties of the power set are :
- The power set of any set is non-empty.
- Each element of a Power set is a set.
- Number of elements in $P(A)=$ Number of subsets of $\operatorname{set} A =2 ^{n(A)}$ where $n(A)$ is the number of elements in set $A$
- It is always larger than the original set.
- The power set of a finite set has a finite number of elements.
- For a set of natural numbers, we can do a one-to-one mapping of the resulting set, $P(S)$, with the real numbers.
- $P(S)$ of set $S$, if operated with the union of sets, the intersection of sets and the complement of sets, denotes the example of Boolean Algebra.
Power Set Examples with Solutions
Working through examples is one of the best ways to understand the concept of a power set. By listing all possible subsets of different sets, we can clearly see how power sets are formed and how their size follows the $2^n$ rule. This section provides step-by-step power set examples with detailed solutions for better clarity.
Power Set of 2-Element Set
Let the original set be $A = \{1, 2\}$.
To find the power set of a 2-element set, we list all possible subsets of $A$, including the empty set and the set itself.
The subsets of $A$ are:
- $\emptyset$
- $\{1\}$
- $\{2\}$
- $\{1, 2\}$
Hence, the power set of $A$ is:
$P(A) = \{\emptyset, \{1\}, \{2\}, \{1, 2\}\}$
The total number of subsets = $2^n = 2^2 = 4$
Power Set of 3-Element Set
Let $B = \{a, b, c\}$
To find the power set of a 3-element set, we again list all possible combinations of elements.
The subsets of $B$ are:
- $\emptyset$
- $\{a\}, \{b\}, \{c\}$
- $\{a, b\}, \{a, c\}, \{b, c\}$
- $\{a, b, c\}$
So, the power set of $B$ is:
$P(B) = \{\emptyset, \{a\}, \{b\}, \{c\}, \{a, b\}, \{a, c\}, \{b, c\}, \{a, b, c\}\}$
Total subsets = $2^3 = 8$
Verifying Total Subsets Using $2^n$
The number of elements in a power set is given by the formula:
$|P(S)| = 2^n$
where $n$ is the number of elements in the original set $S$.
For example, if $S = \{x, y, z, w\}$, then $n = 4$ and:
$|P(S)| = 2^4 = 16$
This formula helps verify whether all subsets have been correctly listed when creating a power set.
Power Set in Mathematics and Real-Life Applications
The concept of a power set extends beyond theoretical math and finds applications in various real-life and academic fields. From logic and programming to probability and combinatorics, power sets help model choices, events, and states. This section explores how power sets are used in both mathematics and practical scenarios.
Power Set in Boolean Algebra and Logic
In Boolean algebra, the power set is often used to represent truth tables and logical operations. Each subset in the power set can correspond to a specific combination of truth values.
For instance, for a set $S = \{p, q\}$ representing propositions, its power set models all possible truth value assignments to $p$ and $q$. This is crucial in designing logical circuits and truth tables.
Use of Power Set in Computer Science and Programming
In computer science, the concept of a power set is essential in various algorithms:
- Generating all possible combinations in recursive or iterative programming
- Bitmasking techniques, where each bit representsthe inclusion/exclusion of an element
For a set with $n$ elements, the power set helps in solving problems involving subset generation, permissions systems, and state spaces.
Power Set in Combinatorics and Probability
In combinatorics, the power set represents the complete collection of subsets, allowing us to count or evaluate combinations.
In probability, if each subset represents a possible event, the power set forms the sample space of all possible events.
For example, if $A = \{\text{Rain}, \text{Snow}\}$, the power set gives us:
$P(A) = \{\emptyset, \{\text{Rain}\}, \{\text{Snow}\}, \{\text{Rain, Snow}\}\}$
Each element of $P(A)$ represents a possible outcome or event in the probability space.
Difference Between Set and Power Set
Understanding the difference between a set and its power set is crucial in set theory. While a set contains individual elements, its power set includes all possible subsets of those elements. This section explains how the two differ in structure, size, and representation.
Element vs Subset
A set contains individual elements, while a power set contains subsets of those elements.
For example:
- Set: $S = {1, 2}$
- Power set: $P(S) = \{\emptyset, {1}, {2}, {1, 2}\}$
In $S$, $1$ is an element. In $P(S)$, $\{1\}$ is a subset.
This highlights the difference between elements and subsets in power sets.
Cardinality Comparison
Let $T$ be a finite set with $n$ elements.
- Cardinality of the set: $|T| = n$
- Cardinality of the power set: $|P(T)| = 2^n$
Example:
If $T = \{a, b, c\}$, then:
- $|T| = 3$
- $|P(T)| = 2^3 = 8$
The power set always has exponentially more elements than the original set. This cardinality difference between a set and its power set is a key concept in set theory and combinatorics.
Solved Examples Based on Power Set
Example 1: Power set of $\{1,2,3\}$ is
1) $P(A)=\{\phi,\{1\},\{2\},\{3\},\{1,2\},\{1,3\},\{2,3\}\}$
2) $P(A)=\{\{1\},\{2\},\{3\},\{1,2\},\{1,3\}\{2,3\},\{1,2,3\}\}$
3) $P(A)=\{\phi,\{1\},\{2\},\{3\},\{1,2\},\{1,3\},\{2,3\},\{1,2,3\}\}$
4) None of the above
Solution:
As we know, in this question,
Option (3) shows all the subsets of $\{1,2,3\}$
Hence, the answer is option 3.
Example 2: Find the number of elements in the power set of $\{1,2,3,4,5\}$.
1) $8$
2) $16$
3) $32$
4) $64$
Solution:
As we know, in this question,
The power set of $\{1,2,3,4,5\}$ will have $2^5=32$ elements.
Hence, the answer is option 3.
Example 3: $\mathbf{A}$ is a set containing $\mathbf{n}$ elements. A subset $P_1$ is chosen and $\mathbf{A}$ is reconstructed by replacing the elements of $P_1$. The same process is repeated for subsets $P_1, P_2, \ldots, P_m$ with $m>1$. The number of ways of choosing $P_1, P_2, \ldots, P_m$, $P_1 \cup P_2 \cup \ldots \cup P_m=A$ so that $P_1 \cup P_2 \cup \ldots \cup P_m=A$ is
1) $\left(2^m-1\right)^{m n}$
2) $\left(2^n-1\right)^m$
3) ${ }^{m+n} C_m$
4) none of these
Solution:
$\operatorname{Let} A=\left\{a_1, a_2, \ldots, a_n\right\}$ for each $a_i(1 \leq i \leq n)$
Either $a_i \in P_j$ or $a_i \notin P_j(1 \leq j \leq m)$
$\therefore$ There are $2^m$ choices in which $a_i, a_j$ belongs to $P_j$,
Also, there is exactly one choice, i.e, $a_i \notin P_j$
$\therefore a_i \in P_1 \cup P_2 \cup \ldots \cup P_m$ in ( $2^m-1$ ) ways.
Since there are n elements in the set A, the number of ways of constructing subsets.
$P_1, P_2, \ldots, P_m$ is $\left(2^m-1\right)^n$
Example 4: The number of elements in the power set of $P(P(\phi))$ is
1) $1$
2) $2$
3) $3$
4) $4$
Solution:
Number of elements in $\mathrm{P}(\phi)$ is $2^0=1$
Number of elements in $\mathrm{P}(\mathrm{P}(\phi))$ is $2^1=2$
Hence, the answer is option 2.
Example 5: A relation on the set $A=\{x:|x|<3, x \in Z\} \quad$ where $\mathbf{Z}$ is the set of integers is defined by $R=\{(x, y): y=|x|, x \neq-1\}$ Then the number of elements in the power set of $\mathbf{R}$ is :
1) 32
2) 16
3) 8
4) 64
Solution:
Consider set $A=\{x:|x|<3, x \in Z\}$.
All elements of the set A, defined by x, are also contained in Z, the set of integers. It's also given that the absolute value of these elements is strictly less than 3.
$|x|<3 \Rightarrow|x| \in\{0,1,2\} \subset A$
Hence, possibly this set in roster form will be,
$A=\{-2,-1,0,1,2\}$
A relation R on the set A where $R \subseteq(A \times A)$ is defined in set builder notation as
$R=\{(x, y): y=|x|, x \neq-1\}$
Thus, $ xRy$ is possible if y is the absolute value of x and x is not equal to -1.
And also, $(x, y) \in(A \times A)$
$|x|=y \in\{0,1,2\}$
$\therefore x \in\{-2,0,1,2\} \quad \text { but } \quad x \neq-1$
Hence, possibly $R$ in roster form will be,
$R=\{(-2,2),(0,0),(1,1),(2,2)\}$
Here we get that $|R|=4$.
Thus,
$|P(R)|=2^{|R|}=2^4=16$
Hence, the answer is option 2.
List of Topics Related to Power Set
To fully grasp the concept of a power set, it is essential to understand the foundational topics of set theory that support it. These related concepts, such as union, intersection, complement, and set representation, form the basis for generating and analysing power sets. In this section, we highlight all the key topics that are directly or indirectly connected to the idea of power sets.
NCERT Resources
Explore essential NCERT study materials for Sets, with comprehensive solutions, concise revision notes, and curated exemplar problems. These resources are designed to enhance your conceptual clarity and prepare effectively for board and competitive exams.
NCERT Solutions for Class 11 Chapter 1 Sets
Practice Questions on Power Sets
Mastering any mathematical concept comes through continuous practice. To help strengthen your understanding of the topic, we have provided some practice questions on Power Set. They will test your knowledge of formulas, important properties and general application of knowledge.
To practice questions based on Power Set Universal Set - Practice Question MCQ click here.
You can practice the next topics of Sets below:
Intersection Of Set Properties Of Intersection - Practice Question MCQ |
Complement Of A Set Law Of Complement Property Of Complement - Practice Question MCQ |
Frequently Asked Questions (FAQs)
The concept of a universal set (a set containing all possible elements) is problematic in standard set theory because its power set would lead to contradictions. This limitation, related to power sets, led to the development of more sophisticated set theories.
Power sets play a crucial role in many set-theoretic paradoxes, such as Russell's paradox. These paradoxes often arise from considering "too large" collections, highlighting the complexities and limitations of naive set theory.
A topology on a set X is defined as a collection of subsets of X (i.e., a subset of the power set of X) that includes both X and the empty set, and is closed under finite intersections and arbitrary unions.
In probability theory, a σ-field (or σ-algebra) is a subset of the power set of the sample space, closed under certain operations. The power set provides the largest possible σ-field for any given sample space.
The power set of any set, equipped with the operations of union, intersection, and complement, forms a Boolean algebra. This provides a concrete example of Boolean algebras, which are fundamental in logic and computer science.
While a power set contains all possible subsets of a set, a partition is a specific subset of the power set where the subsets are non-empty, mutually exclusive, and their union is the entire set.
In topology, a topology on a set X is defined as a subset of the power set of X that satisfies certain axioms. The power set itself is always a valid topology, known as the discrete topology.
In fuzzy set theory, the power set concept is extended to fuzzy power sets, where each element in the power set has a degree of membership between 0 and 1, rather than just being present or absent.
The power set of any set, when ordered by set inclusion, forms a complete lattice. This connection is fundamental in order theory and provides a concrete example of a Boolean algebra.
The power set of any set forms a Boolean ring under the operations of symmetric difference (as addition) and intersection (as multiplication). This connection bridges set theory and abstract algebra.