Difference of Set: Definition, Formula, Properties, Examples
The difference between the two collections is a concept that examines the presence of elements in one collection but not in another. For instance, consider two groups of employees in a company; In one group, some members participated in a mandatory training session, but the other group did not participate in it at all. Therefore, the possibilities in the second set that are not in the first set indicate the number of employees who have not experienced the training. This operation is essential for defining the specific problem and issue domains or domains that require a solution in specific sectors or segments within a broad classification.
- What is Difference of sets?
- Properties of Difference of Sets
- Symmetric Difference of Sets $(A Δ B)$
- Solved Examples Based On the Difference of Sets
In this article, we will cover the concept of the difference of sets. This concept falls under the broader category of sets relation and function, a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of one question has been asked on this concept, including one in 2021.
What is Difference of sets?
Difference of sets is one of the fundamental operations in the concept of sets. It has various applications in concepts involving a specific category. Before looking into the concept of difference of sets, let us see what are sets.
Set
Sets are a foundational concept in mathematics, central to various fields such as statistics, geometry, and algebra. A set is simply a collection of distinct objects, considered as a whole. These objects, called elements or members of the set, can be anything: numbers, people, letters, etc. Sets are particularly useful in defining and working with groups of objects that share common properties.
It is a well-defined collection of distinct objects and it is usually denoted by capital letters A, B, C, S, U, V…...
Difference of Sets Definition
The difference of sets $A$ and $B$ in this order is the set of elements that belong to $A$ but not to B.
Symbolically, we write $A - B$ and read as "A minus B".
How to find the difference of sets?
To find the difference of sets,
- Identify the given non-empty sets and write them in set-builder form.
- Identify the order of difference, i.e., if to find $A- B$ or $B - A$.
- Find the intersection of the sets $A$ and $B$.
- Eliminate the elements of $A \cap B$ from Set $A$ to write a new set. In other words, remove the elements in $A$ that are also present in Set $B$.
- Define the new set as $A-B$. Here, $A-B$ is the set of elements of $A$ that are not in $B$.
Now, let us look into difference of set example for better understanding.
Difference of Set Examples
1. $A=\{1,2,3,4\}$ and $B=\{4,5,6,8\}$,
then, $A-B=\{1,2,3\}$ and $B-A=\{5,6,8\}$
2. $A = \{blue, violet, green, yellow, black, white, red\}$ and $B = \{black, blue, red, grey, brown\}$, then $A-B=\{violet, green, yellow, white\}$ and $B-A = \{grey, brown\}$
3. $A = \{x: x \in \mathbf N$ and $x \leq 10\}$ and $ B=$ set of all integers greater than $-5$ and less than or equal to $5$.
Now, $A = \{1,2,3,4,5,6,7,8,9\}$ and $B = \{-4,-3,-2,-1,0,1,2,3,4,5\}$, then $A-B=\{6,7,8,9\}$ and $B-A =\{-4,-3,-2,-1,0\}$
4. $A=$ set of all even natural numbers less than $10$ and $B=\{2,4,6,8\}$. Now, $A = \{2,4,6,8\}$ and $B=\{2,4,6,8\}$, then $A-B=\phi$ and $B-A =\phi$
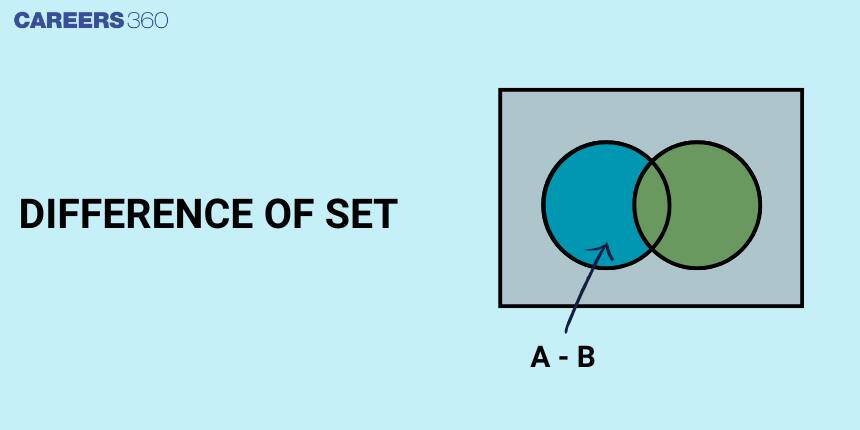
Difference of Set Venn Diagram
The difference of sets $A$ and $B$ is $A-B$. The difference of set Venn diagram is

For instance, The difference of sets venn diagram of $A=\{1,2,3,4\}$ and $B=\{4,5,6,8\}$ is

From the venn diagram, $A-B=\{1,2,3\}$ and $B-A=\{5,6,8\}$.
Properties of Difference of Sets
1. In general $A - B$ does not equal $B - A$. This means that the difference of sets are not interchangeable.
2. When we take the difference of the same set then the result is empty. (i.e) $\mathrm{A}-\mathrm{A}=\phi$
3. When we take the difference of a finite set with the null set then the result is a finite set. (i.e) $\mathrm{A}-\phi=\mathrm{A}$
4. When we take the difference of a finite set with a universal set then the result is empty. (i.e) $A-U=\phi$
5. The sets $A-B, A \cap B$ and $B-A$ are mutually disjoint sets, (i.e) the intersection of any two of these sets is the null set(empty set).
Symmetric Difference of Sets $(A Δ B)$
Symmetric difference of two sets $A$ and $B$ is defined as $A Δ B = ( A - B ) ∪ ( B - A )$. This can also be represented as $A Δ B = ( A ∪ B) - ( A ∩ B )$
The symmetric difference of sets can be found with the help of the following steps:
- Identify the given non-empty sets and write them in set-builder form.
- Now find the difference $A-B$ and $B-A$.
- Now find the union of those sets $A-B$ and $B-A$.
Symmetric Difference of Sets Examples
1. $A=\{1,2,3,4\}$ and $B=\{4,5,6,8\}$,
then, $A-B=\{1,2,3\}$ and $B-A=\{5,6,8\}$. The symmetric difference of set are $(A-B) \cup (B-A) = \{1,2,3\} \cup \{5,6,8\} = \{1,2,3,5,6,8\}$
2. $A = \{blue, violet, green, yellow, black, white, red\}$ and $B = \{black, blue, red, grey, brown\}$, then $A-B=\{violet, green, yellow, white\}$ and $B-A = \{grey, brown\}$. The symmetric difference of set are $(A-B) \cup (B-A) = \{violet, green, yellow, white\} \cup \{grey, brown\} = \{violet, green, yellow, white, grey, brown\} $
3. $A = \{x: x \in \mathbf N$ and $x \leq 10\}$ and $ B=$ set of all integers greater than $-5$ and less than or equal to $5$.
Now, $A = \{1,2,3,4,5,6,7,8,9\}$ and $B = \{-4,-3,-2,-1,0,1,2,3,4\}$, then $A-B=\{5,6,7,8,9\}$ and $B-A =\{-4,-3,-2,-1,0\}$. The symmetric difference of set are $(A-B) \cup (B-A) = \{5,6,7,8,9\} \cup \{-4,-3,-2,-1,0\} = \{-4,-3,-2,-1,0,5,6,7,8,9\}$
4. $A=$ set of all even natural numbers less than $10$ and $B=\{2,4,6,8\}$. Now, $A = \{2,4,6,8\}$ and $B=\{2,4,6,8\}$, then $A-B=\phi$ and $B-A =\phi$. The symmetric difference of set are $(A-B) \cup (B-A) = \phi \cup \phi = \phi$
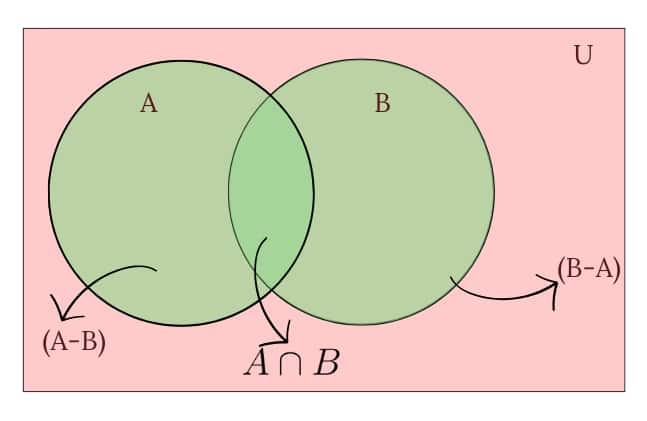
Symmetric Difference of Set Venn Diagram
The symmetric difference of sets $A$ and $B$ is $(A-B) \cup (B-A)$. The symmetric difference of set Venn diagram is

For instance, The difference of sets venn diagram of $A=\{1,2,3,4\}$ and $B=\{4,5,6,8\}$ is

From the venn diagram, $A Δ B = \{1,2,3,5,6,8\}$
Recommended Video Based on Difference of Sets
Solved Examples Based On the Difference of Sets
Example 1: If $\mathbf{A}, \mathbf{B}$, and $\mathbf{C}$ are non-empty sets, then $(A \cup B)-(A \cap B)$
1) $(A \cup B)-B$
2) $A-(A \cap B)$
3) $(A-B) \cup(B-A)$
4) $(A \cap B) \cup(A \cup B)$
Solution:
Clearly, as the sets in the question and in the third option, both equal the symmetric difference of $A$ and $B$, so both these are equal.
$
(A-B) \cup(B-A)=(A \cup B)-(A \cap B)
$
Hence, the answer is the option 3.
Example 2: $
\begin{aligned}
& \mathrm{A}=\left\{\mathrm{n} \in \mathrm{N} \mid \mathrm{n}^2 \leq \mathrm{n}+10,000\right\}, \mathrm{B}=\{3 \mathrm{k}+1 \mid \mathrm{k} \in \mathrm{N}\} \\
& C=\{2 \mathrm{k} \mid \mathrm{k} \in \mathrm{N}\} A \cap(B-C)
\end{aligned}
$
$ \text { Let } \mathrm{A}=\left\{\mathrm{n} \in \mathrm{N} \mid \mathrm{n}^2 \leq \mathrm{n}+10,000\right\}, \mathrm{B}=\{3 \mathrm{k}+1 \mid \mathrm{k} \in \mathrm{N}\} \text { and } C=\{2 \mathrm{k} \mid \mathrm{k} \in \mathrm{N}\} \text {, then the sum of all the elements of the set } A \cap(B-C) \text { is equal to } $
Solution:
$\begin{aligned} & A: n^2-n \leq 10,000 \\ & \Rightarrow n(n-1) \leq 100 \cdot 100 \\ & \Rightarrow n=\{1,2, \ldots, 100\} \\ & B=\{4,7,10,13, \ldots\} \\ & C=\{2,4,6,8,10, \ldots\} \\ & B-C=\{7,13,19, \ldots\} \\ & \begin{aligned} A \cap(B-C)=\{7,13,19, \ldots, 97\} \\ \text { Sum }=\frac{16}{2}\{14+14.6\} \\ \quad=832\end{aligned}\end{aligned}$
Hence, the answer is 832.
Example 3:
Let $\mathrm{A}, \mathrm{B}$, and $\mathbf{C}$ be sets such that $\phi \neq A \cap B \subseteq C$. Then which of the following statements is not true?
1) $B \cap C \neq \phi$
2) If $(A-B) \subseteq C$, then $A \subseteq C$
3) $(C \cup A) \cap(C \cup B)=C$
4) If $(A-C) \subseteq B$, then $A \subseteq B$
Solution:
$
\begin{aligned}
& \text { As }(A \cap B) \subseteq C \\
& \Rightarrow(A \cap B) \subseteq(B \cap C) \\
& \text { as }(A \cap B) \neq \phi_{\Rightarrow}(B \cap C) \neq \phi
\end{aligned}
$
So, option (1) is true
Let $x \in A$ and $x \notin B \Rightarrow x \epsilon(A-B)_{\Rightarrow} \Rightarrow x \epsilon C$ let $x \in A$ and $x \epsilon B \Rightarrow x \epsilon(A \cap B) \Rightarrow x \epsilon C$ Hence $x \in A$ and $x \in C \Rightarrow A \subseteq C$ So, option (2) is true.
Let
$\begin{aligned} & x \in C, x \in(C \cup A) \cap(C \cup B)) \\ & =>x \epsilon(C \cup A) \text { and } x \epsilon(C \cup B)) \\ & (x \in C \text { or } x \in A) \text { and }(x \in C \text { or } x \in B) \\ & =>x \epsilon C \text { or } x \epsilon(A \cap B) \\ & =>x \epsilon C \quad A s, A \cup B \subseteq C\end{aligned}$
$\begin{aligned}
&(C \cup A) \cap(C \cup B) \subseteq C.......\text { (1) }
\end{aligned}$
Now,
$
\begin{aligned}
& =>x \epsilon C \\
& =>x \epsilon(C \cup A) \cap(C \cup B) \\
& =>C \subseteq(C \cup A) \cap(C \cup B) ......(2)
\end{aligned}
$
From (1) and (2)
$
(C \cup A) \cap(C \cup B)=C
$
=> Option (3) is correct.
For $\mathrm{A}=\mathrm{c}, A-C=\phi$
$
=>\phi \subseteq B \text { but }=>A \nsubseteq B
$
So, option (4) is not true.
Example 4:
If $A=(x \in \mathbf{R}:|x|<2)$ and $B=(x \in \mathbf{R}:|x-2| \geqslant 3)$; then:
1) $A-B=[-1,2)$
2) $B-A=\mathbf{R}-(-2,5)$
3) $A \cup B=\mathbf{R}-(2,5)$
4) $A \cap B=(-2,-1)$
Solution:
$\begin{aligned} & A=\{x: x \in(-2,2)\} \\ & B=\{x: x \in(-\infty,-1] \cup[5, \infty)\} \\ & A \cap B=\{x: x \in(-2,-1]\} \\ & A \cup B=\{x: x \in(-\infty, 2) \cup[5, \infty)\} \\ & A-B=\{x: x \in(-1,2)\} \\ & B-A=\{x: x \in(-\infty,-2] \cup[5, \infty)\}\end{aligned}$
Hence, the answer is the option 2.
List of Topics Related to Difference of Sets
Frequently Asked Questions (FAQs)
The difference of set definition is, $A-B$ in this order is the set of elements that belong to $A$ but not to $B$.
The intersection of any two of these sets is the null set is called a disjoint set.
The symmetric difference of two sets $A$ and $B$ is defined as
$A \Delta B=(A-B) \cup(B-A)$. The symmetric difference of set A and B can also represented as $A Δ B = ( A ∪ B) - ( A ∩ B )$
The set difference of set A with null set is $A-\{\} = A$.
No, $A-B$ is not equal to $B-A$.
The difference of sets, also known as set difference or relative complement, is an operation that results in a new set containing elements that are in one set but not in another. It's denoted by A - B or A \ B, where A is the set from which we're subtracting and B is the set being subtracted.
A - B and B - A are generally not the same. A - B contains elements that are in A but not in B, while B - A contains elements that are in B but not in A. These sets can be different unless A and B are identical or one is a subset of the other.
The formula for the difference of sets A and B is:
Yes, the difference of two sets can be an empty set. This occurs when all elements of the first set are also present in the second set, or when the first set is a subset of the second set. For example, if A = {1, 2, 3} and B = {1, 2, 3, 4, 5}, then A - B = ∅ (empty set).
Set difference and set complement are related but distinct concepts. The difference A - B gives elements in A but not in B. The complement of A, denoted A', gives all elements in the universal set that are not in A. If B is the universal set, then A - B is equivalent to the complement of A.
Set difference is related to other set operations through various identities. For example:
The symmetric difference of sets A and B, denoted A Δ B, is the set of elements that are in either A or B, but not in both. It can be expressed using set difference as:
No, set difference is not associative. This means that (A - B) - C is not necessarily equal to A - (B - C). For example, if A = {1, 2, 3}, B = {2, 3, 4}, and C = {3, 4, 5}, then:
The cardinality of the difference of sets A and B is always less than or equal to the cardinality of A. Mathematically:
A - B and A - (A ∩ B) are equivalent. Both expressions result in the set of elements that are in A but not in B. The intersection A ∩ B represents the common elements of A and B, so subtracting this from A gives the same result as directly subtracting B from A.
Yes, you can perform set difference with more than two sets, but it's typically done sequentially. For example, A - B - C is interpreted as (A - B) - C. This is because set difference is not associative, so the order of operations matters.
The power set difference refers to the difference operation applied to power sets. If P(A) and P(B) are the power sets of A and B respectively, then P(A) - P(B) is the set of all subsets of A that are not subsets of B.
If A is a subset of B (A ⊆ B), then A - B = ∅ (empty set). Conversely, if A - B = ∅, then A must be a subset of B. This relationship helps in understanding subset relationships between sets.
The absolute complement of a set A, denoted A', is the set of all elements in the universal set that are not in A. The relative complement, or set difference A - B, is the set of elements in A that are not in B. The absolute complement is a special case of relative complement where B is the universal set.
Set difference can be applied to infinite sets just as it is to finite sets. The result may be finite or infinite depending on the nature of the sets involved. For example, the difference between the set of all integers and the set of even integers is the set of all odd integers, which is infinite.
Set difference corresponds to the logical operation of conjunction (AND) with negation. For sets A and B, the statement "x is in A - B" is equivalent to the logical statement "x is in A AND x is not in B". This connection helps in understanding set operations in terms of logical propositions.
In a Venn diagram, the difference A - B is represented by the region that is inside circle A but outside circle B. It's typically shaded or highlighted to show the elements unique to A. This visual representation helps in understanding the concept intuitively.
Set difference and set subtraction are the same operation. The terms are used interchangeably in set theory. Both refer to the operation of creating a new set containing elements from one set that are not in another set.
If sets A and B are disjoint (have no common elements), then A - B = A and B - A = B. In other words, subtracting a disjoint set has no effect. This property highlights the relationship between set difference and the overlap (or lack thereof) between sets.
No, the set difference A - B cannot result in a set larger than A. The difference operation can only remove elements from A, never add to it. Therefore, |A - B| ≤ |A| for any sets A and B.
The empty set ∅ is the identity element for set difference. For any set A, A - ∅ = A. However, note that ∅ - A = ∅ for any set A, so the identity property only holds when the empty set is the second operand.
Set difference can be used to test for set equality. If A - B = ∅ and B - A = ∅, then A = B. This is because if neither set has any elements that the other doesn't, they must contain exactly the same elements.
Set difference can be used to create partitions of a set. If A is partitioned into subsets B and C, then B = A - C and C = A - B. This relationship shows how set difference can be used to divide a set into mutually exclusive subsets.
For any set A in a universal set U, A - U = ∅ (empty set), because there are no elements in A that are not in U. Conversely, U - A = A' (the complement of A), as it contains all elements in the universal set that are not in A.
The double difference property states that (A - B) - C = A - (B ∪ C). This property shows how repeated set difference operations can be simplified using union. It's useful in simplifying complex set expressions.
If A is a proper subset of B (A ⊂ B), then B - A is non-empty. This non-empty difference contains the elements that make B a larger set than A. Conversely, if B - A is non-empty and A ⊆ B, then A is a proper subset of B.
Set difference A - B includes elements in A but not in B, while symmetric difference A Δ B includes elements in either A or B, but not in both. Symmetric difference can be expressed as (A - B) ∪ (B - A), combining both one-way differences.
Set difference distributes over intersection but not over union. That is:
De Morgan's laws can be applied to set difference. The complement of A - B can be expressed as:
If A ⊆ B ⊆ C, then:
In probability theory, the set difference can be used to calculate the probability of an event A occurring but not event B. If P(A) is the probability of A and P(A ∩ B) is the probability of both A and B occurring, then P(A - B) = P(A) - P(A ∩ B).
In database systems, set difference is often used in queries to find records that exist in one table but not in another. This operation is typically implemented using the MINUS or EXCEPT keyword in SQL, allowing for complex data filtering and comparison.
Symmetric difference is defined using set difference. For sets A and B, the symmetric difference A Δ B can be expressed as:
If events A and B are mutually exclusive, then A ∩ B = ∅. In this case, A - B = A and B - A = B. This property of set difference can be used to verify or define mutual exclusivity in probability and set theory.
For any set A, A - A = ∅ (empty set). This property demonstrates that a set has no elements that are not in itself, and it's a fundamental identity in set theory. It's analogous to the arithmetic property that any number minus itself equals zero.
The axiom of extensionality states that two sets are equal if and only if they have the same elements. Set difference can be used to test this: if A - B = ∅ and B - A = ∅, then A and B have the same elements and are therefore equal according to this axiom.
In set theory, the universe (or universal set) U contains all elements under consideration. For any set A in this universe, U - A gives the complement of A. This relationship shows how set difference can be used to define complementation relative to a given universe.
Set equality can be defined using set difference: two sets A and B are equal if and only if both A - B and B - A are empty sets. This definition captures the idea that equal sets contain exactly the same elements, with none unique to either set.
Set difference can be used to define proper subsets: A is a proper subset of B if and only if A ⊆ B and B - A ≠ ∅. This definition captures the idea that a proper subset is contained within another set but is not equal to it.
The power set of A, denoted P(A), is the set of all subsets of A. The difference between power sets, P(A) - P(B), gives all subsets of A that are not subsets of B. This operation can be used to compare the structural relationships between sets.
The expression (A - B) ∩ B always equals the empty set ∅. This is because A - B contains elements that are not in B, while B contains only elements in B. Their intersection is thus empty, illustrating a fundamental property of set difference.
Set difference preserves countability: if A is countable and B is any set, then A - B is also countable. However, if A is uncountable and B is countable, A - B remains uncountable. This property is important in understanding the cardinality of sets resulting from difference operations.
In Boolean algebra, the complement of a set A is equivalent to U - A, where U is the universal set. This connection shows how set difference in set theory relates to complementation in Boolean algebra, bridging these two mathematical areas.
Two sets A and B are disjoint if and only if A - B = A and B - A = B. This definition using set difference captures the idea that disjoint sets have no elements in common, as subtracting one from the other has no effect.
Set difference is crucial in defining the domain and range of functions in set theory. For a function f: A → B, the effective domain of f can be defined as A - {x ∈ A | f(x) is undefined}, helping to precisely specify the set of valid inputs for the function.
In the construction of quotient sets, set difference can be used to define equivalence classes. If ~ is an equivalence relation on a set A, then for any a ∈ A, its equivalence class [a] can be thought of as A - {x ∈ A | x ≁ a}, where ≁ denotes "not equivalent to".
The expression (A - B) ∪ (B - A) is the definition of the symmetric difference of A and B, often denoted A Δ B. This operation gives all elements that are in either A or B, but not in both, highlighting how set difference can be used to construct more complex set operations.
In topology, set difference is used to define closed sets. If X is a topological space and A is a subset of X, then X - A is open if and only if A
Also Read
21 Jul'25 11:37 AM
02 Jul'25 07:43 PM
02 Jul'25 07:42 PM
02 Jul'25 06:39 PM
02 Jul'25 06:39 PM
02 Jul'25 06:38 PM
02 Jul'25 06:38 PM
02 Jul'25 06:38 PM
02 Jul'25 06:38 PM
02 Jul'25 06:38 PM